The pre-calculus final exam cheat sheet provides a concise and comprehensive overview of the key concepts and formulas covered in a typical pre-calculus course. This cheat sheet is an invaluable resource for students preparing for their final exam, offering quick access to essential information and formulas.
From functions and graphs to derivatives and integrals, this cheat sheet covers a wide range of topics, providing students with the tools they need to succeed on their exam.
Functions and Graphs
In pre-calculus, the study of functions and their graphs is essential for understanding a wide range of mathematical concepts. Different types of functions exhibit distinct characteristics, and being able to recognize and graph them is a crucial skill.
To effectively graph a function, it is important to first identify its key features, including the domain, range, intercepts, and asymptotes. The domain represents the set of all possible input values for the function, while the range represents the set of all possible output values.
Intercepts are points where the graph intersects the x- or y-axis, and asymptotes are lines that the graph approaches but never touches.
Types of Functions
- Linear functions:Represented by equations of the form y = mx + b, where m is the slope and b is the y-intercept. They produce straight lines on a graph.
- Quadratic functions:Represented by equations of the form y = ax² + bx + c, where a, b, and c are constants. They produce parabolas on a graph.
- Polynomial functions:Represented by equations of the form y = a nx n+ a n-1x n-1+ … + a 1x + a 0, where a n, a n-1, …, a 1, and a 0are constants. They produce curves with varying shapes depending on the degree of the polynomial.
- Exponential functions:Represented by equations of the form y = a x, where a is a positive constant. They produce curves that increase or decrease rapidly.
- Logarithmic functions:Represented by equations of the form y = log ax, where a is a positive constant. They produce curves that are the inverse of exponential functions.
- Trigonometric functions:Represented by equations of the form y = sin(x), y = cos(x), y = tan(x), and so on. They produce periodic curves that repeat over a certain interval.
Trigonometry
Trigonometry is a branch of mathematics that deals with the relationships between the sides and angles of triangles. It is used in a wide variety of applications, including surveying, navigation, and engineering.
The Unit Circle
The unit circle is a circle with radius 1. It is used to define the trigonometric functions.
The unit circle is divided into four quadrants. The first quadrant is the quadrant in which the x- and y-coordinates are both positive. The second quadrant is the quadrant in which the x-coordinate is negative and the y-coordinate is positive.
The third quadrant is the quadrant in which the x- and y-coordinates are both negative. The fourth quadrant is the quadrant in which the x-coordinate is positive and the y-coordinate is negative.
Trigonometric Functions
The trigonometric functions are functions that are defined in terms of the coordinates of a point on the unit circle.
The six trigonometric functions are:
- Sine (sin)
- Cosine (cos)
- Tangent (tan)
- Cosecant (csc)
- Secant (sec)
- Cotangent (cot)
The trigonometric functions can be used to find the lengths of sides and the measures of angles in triangles.
Solving Trigonometric Equations
Trigonometric equations are equations that involve trigonometric functions.
There are a variety of methods that can be used to solve trigonometric equations.
- Factoring
- Using the trigonometric identities
- Using a graphing calculator
Limits and Continuity
Limits and continuity are fundamental concepts in calculus that describe the behavior of functions as their inputs approach specific values. Understanding these concepts is essential for studying higher-level mathematics and its applications.
Definition of Limits
The limit of a function f(x) as x approaches a value c, denoted as lim_(x->c) f(x), represents the value that the function approaches as x gets closer and closer to c. Formally, it is defined as:
lim_(x->c) f(x) = L if for any ε > 0, there exists a δ > 0 such that if 0< |x - c| < δ, then |f(x) - L| < ε.
Evaluating Limits
There are various techniques for evaluating limits, including:
- Substitution: If the limit exists, it can be found by simply substituting the value of c into the function.
- Factoring and canceling: This method can be used to simplify the function and make the limit easier to evaluate.
- L’Hopital’s rule: This rule is used to evaluate limits of indeterminate forms, such as 0/0 or ∞/∞.
Continuity
A function f(x) is continuous at a point c if it satisfies the following conditions:
- f(c) exists.
- lim_(x->c) f(x) exists.
- lim_(x->c) f(x) = f(c).
Geometrically, a function is continuous at a point if its graph has no break or jump at that point.
Types of Discontinuities
There are three main types of discontinuities:
- Removable discontinuity: Occurs when the function is undefined at a point but can be made continuous by redefining the function at that point.
- Jump discontinuity: Occurs when the limit of the function exists but is not equal to the value of the function at the point.
- Infinite discontinuity: Occurs when the limit of the function does not exist.
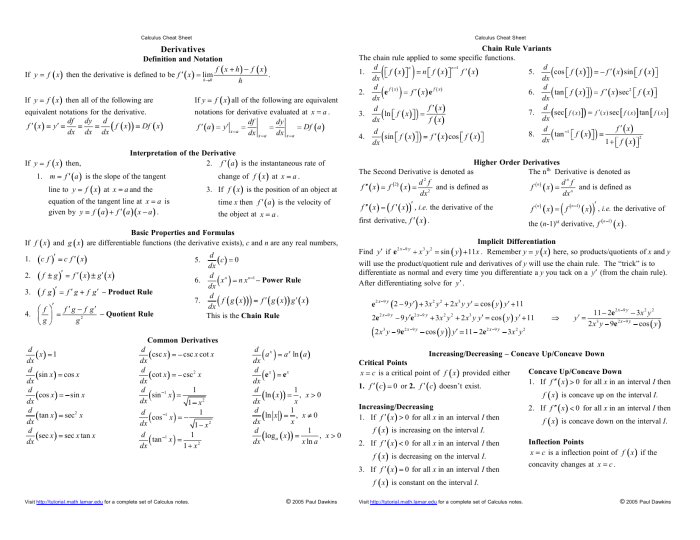
Derivatives: Pre-calculus Final Exam Cheat Sheet
The derivative of a function measures the instantaneous rate of change of the function with respect to its input. It is a fundamental concept in calculus with numerous applications in various fields.
To find the derivative of a function f(x), we use the limit definition:
$$f'(x) = \lim_h\to 0 \fracf(x+h)
f(x)h$$
There are several rules for differentiation, including the power rule, product rule, quotient rule, and chain rule. These rules allow us to find the derivatives of complex functions by breaking them down into simpler components.
Applications of Derivatives
Derivatives have numerous applications, such as:
- Finding critical points (local maxima, minima, and points of inflection)
- Determining the concavity of a function
- Solving optimization problems
- Analyzing the behavior of functions
Integrals
The integral of a function is a mathematical operation that calculates the area under the curve of the function. It is used to find the area of a region, the volume of a solid, and the length of a curve.To
find the integral of a function, we use the following formula:$$\int f(x) dx = F(x) + C$$where \(f(x)\) is the function, \(F(x)\) is the antiderivative of \(f(x)\), and \(C\) is the constant of integration.There are several different rules for integration, including the power rule, the product rule, and the chain rule.
These rules can be used to find the integral of any function.Integrals have a wide range of applications in mathematics and science. They are used to find the area of a region, the volume of a solid, and the length of a curve.
They are also used to solve differential equations and to find the work done by a force.
Applications of Integrals
One of the most important applications of integrals is to find the area of a region. The area of a region can be found by integrating the function that represents the height of the region over the interval that represents the width of the region.Another
important application of integrals is to find the volume of a solid. The volume of a solid can be found by integrating the function that represents the cross-sectional area of the solid over the interval that represents the height of the solid.Integrals
can also be used to find the length of a curve. The length of a curve can be found by integrating the function that represents the arc length of the curve over the interval that represents the length of the curve.
Applications of Pre-Calculus
Pre-calculus is a foundational subject that provides a solid understanding of mathematical concepts and techniques. Its applications extend far beyond the classroom, reaching into various fields such as physics, engineering, economics, and even everyday life.
In physics, pre-calculus is essential for understanding the motion of objects, the behavior of waves, and the interactions between forces. Engineers use pre-calculus to design and analyze structures, machines, and systems, while economists employ it to model economic behavior, analyze market trends, and make predictions.
Real-World Problem Solving
Pre-calculus empowers students to solve real-world problems by providing them with the tools to analyze and interpret data, create models, and make informed decisions. For instance, pre-calculus can be used to:
- Calculate the trajectory of a projectile
- Determine the optimal dimensions of a storage container
- Forecast future economic trends based on historical data
Preparation for Higher-Level Mathematics, Pre-calculus final exam cheat sheet
Moreover, pre-calculus serves as a gateway to higher-level mathematics courses such as calculus, linear algebra, and differential equations. It provides students with the necessary foundation to grasp abstract concepts and develop problem-solving skills that are crucial for success in these advanced subjects.
General Inquiries
What is the purpose of a pre-calculus final exam cheat sheet?
A pre-calculus final exam cheat sheet is a concise and comprehensive overview of the key concepts and formulas covered in a typical pre-calculus course. It provides students with quick access to essential information and formulas, helping them prepare for their final exam.
What topics are covered on the pre-calculus final exam cheat sheet?
The pre-calculus final exam cheat sheet covers a wide range of topics, including functions and graphs, trigonometry, limits, continuity, derivatives, and integrals.
How can I use the pre-calculus final exam cheat sheet?
The pre-calculus final exam cheat sheet is best used as a study aid. It can be reviewed before the exam to refresh your memory on key concepts and formulas. It can also be used during the exam to quickly reference information as needed.




